MAMT 574 Origami in Mathematics and Education

Welcome! If you're looking at this web page, then you're either registered for or planning/thinking of registering for the class MAMT 574 Origami in Mathematics and Education for the Winter 2019 term at Western New England University.

Here you will find some brief information about the course. More details will be provided in the course syllabus. Please feel free to EMAIL ME if you have any questions!

Textbook: None. However, I will be handing out occasional readings, and much of what we do will follow things in the book Project Origami: Activities for Exploring Mathematics, 2nd edition by Thomas Hull, A K Peters, 2012. But buying that book is NOT necessary for the course.

Meeting times: Mondays and Wednesdays from 4:00 - 5:50pm, Jan. 14 - March 25, 2019.

Classroom: Herman Hall room 304.

Instructor: Tom Hull, Associate Professor of Mathematics, WNE. Office: Herman 308-G

Description: Origami, the art of paper folding, has strong connections with mathematics.  The process of folding paper can be explored and modeled with geometry, algebra, number theory, combinatorics, graph theory,  calculus, linear algebra, and many other branches of math.  This makes origami a perfect medium by which math teachers of all levels can infuse hands-on, fun, paper folding explorations into their classes.   In this course we will examine the many ways in which origami can be studied mathematically, giving us a chance to see how many different branches in math can come together around one subject.  We will also pay special attention how such origami projects can be used in middle-school and high-school math classes.  This will be a very hands-on class, with many opportunities for participants to fold things for themselves and prove the math behind the folds. 3 cr. (CORE)

Paper: The instructor will provide origami paper to everyone (funded from an NSF grant) for folding during class and for some homework assignments. However, you may choose to purchase more paper on your own. Good paper to use in this class is: A box of 500 sheets of square memo cube paper (cost: about $3 at any Staples) and 6 inch origami paper (cost: about $6 at any Michael's or art supply store). But do not go buying paper until you receive the free paper the instructor will provide. After that you may decide to go crazy if you like.

Topics covered: As the course description states, we will be using many different brances of math in this course. Since everyone has different backgrounds, this will require reviewing some basic material in-class. However, the subjects we will be making the most use of will be the following:

• Geometry (standard Euclidean geometry, like similar triangles, as well as analytic geometry, using equations of lines and parabolas and such)
• Graph Theory (studying 3D polyhedra as planar graphs is very useful, and we will pay particular attention to graph drawing, cycles on graphs, and graph colorings)
• Combinatorics (suprisingly, a lot can be learned about paper folding by doing things like counting the number of mountain creases and valley creases in a folded object's crease pattern, so combinatorics is a very good tool to use)
• Applications Origami is being used to deploy solar panels in outer space, to design better air bags for cars, and to even make heart stents. In fact, the instructor of this course (Tom) is working with some physicists at UMass-Amherst to apply origami to soft-matter polymers, with hopes of creating new biomedical devices (for example). We'll explore these applications, and maybe even take a field trip to see applied origami in action at UMass.
• Other stuff! (We will see some matrices. We will see some number theory. We might even use some calculus, complex numbers, or abstract algebra! All of these topics will be reviewed as they are introduced.)

Grading: Your grade for the class will be determined by the following:

• 25% Midterm exam
• 25% Final exam (Part of the final be a presentation!)
• 25% Homework
• 25% Taking notes (every class there will be an assigned note-taker (either 1 or 2 people) who will write up a good set of notes for that day's class and submit them to me, which I will then grade, edit, and distribute to the rest of the class, thus creating an official set of class notes)

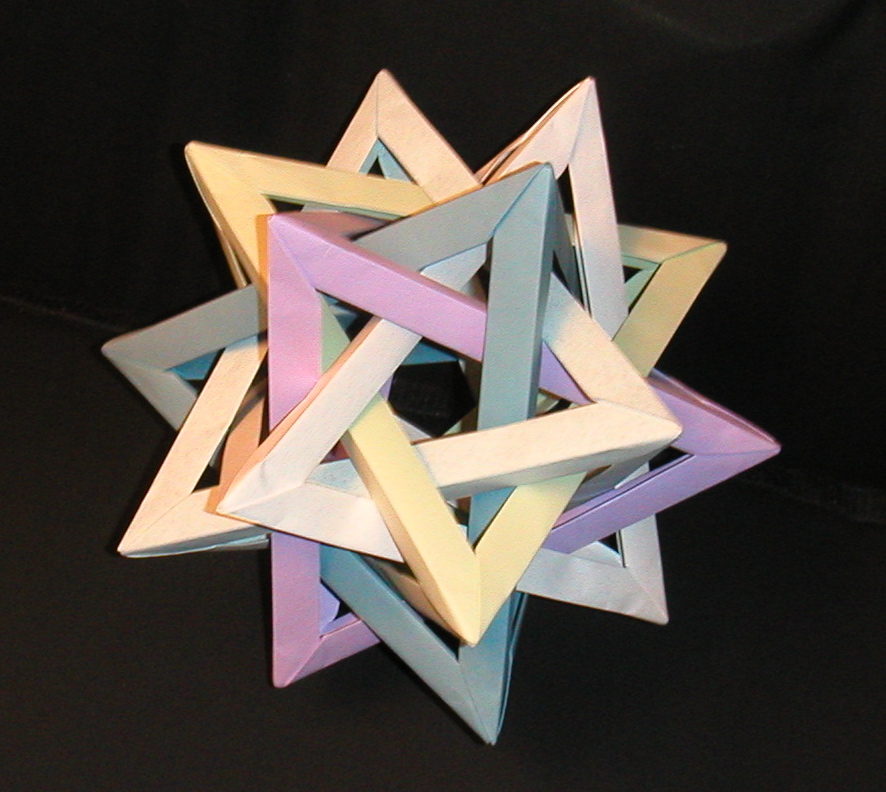
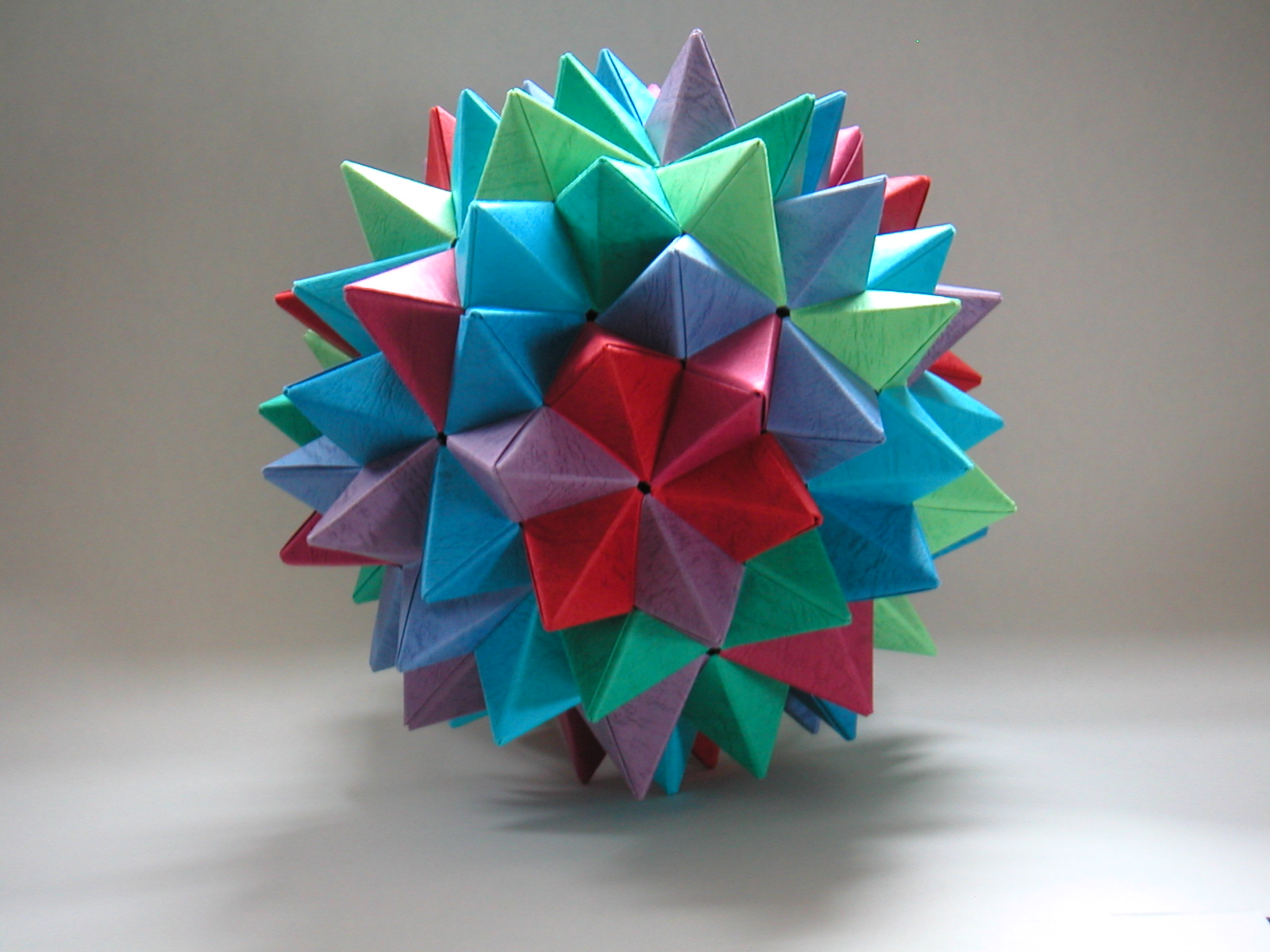
About Folding: There will be a fair amount of paper folding done in class and sometimes for homework. Please do NOT be worried about your own personal paper-folding skill. Most of what we will fold will be at the simple level (although they will use math!) and not complex bugs like the one shown above. However, we will study the math behind such complex-level origami. Those students who do wish to explore such advanced paper folding will have opportunities to do so.

About the instructor: Thomas Hull has been practicing origami since he was 8 years old. He has co-authored two origami instruction books (Origami, Plain and Simple with Robert Neale (1994), and Russian Origami with Sergei Afonkin (1997), both published by St. Martin's Press) and has invented numerous origami models. His PHiZZ Unit has been especially popular, and his Five Intersecting Tetrahedra model was voted by the British Origami Society as one of the top 10 origami models of all time. From 1994-2007 he was on the board of directors for OrigamiUSA, a national nonprofit organization for the advancement of origami. He is also one of the world experts on the mathematics of origami. He edited the book Origami³ (the Proceedings of the Third International Meeting of Origami in Science, Mathematics, and Education) and authored the text for this course, Project Origami. He has been invited to lecture on the mathematics of origami across the USA as well as in Peurto Rico, Europe, and Japan. In other words, this is his specialty and this will be an awesome class!

Links: There are a lot of great resources for origami, and origami-math, online. Below are a few links to explore (feel free to search for more) if you want to get a feel for what origami is all about.

• Robert Lang's web page, which contains pictures of fantastic models as well as information on origami applications in math and science.
• My origami math pages, which are some of the oldest origami-math pages on the web! There are instructions for some of my models as well as previews of some things we'll see in class.
• The web page of OrigamiUSA, which has all sorts of resources for matters origami.